Graphene, a form of pure carbon that exists in one-atom-thick sheets, is of great interest to scientists and engineers because of its strength and other remarkable properties. Graphene sheets can be formed into other structures. The mechanical properties of a graphene nanostructure are different than structures in the macroscale.
A graphene tube with a radius of less than one nanometer is known as a carbon nanotube. MSI Principal Investigator Traian Dumitrica, a professor in the Department of Mechanical Engineering (College of Science and Engineering), has been studying the mechanical properties of carbon nanotubes for several years. In a recent paper that appeared in Physical Review B, Professor Dumitrica and his colleagues modeled bent graphene as a large-radius (> 1nm) carbon nanotube to investigate its bending rigidity. The authors developed a simple analytic formula for the bending energy, which was confirmed by tight-binding objective molecular dynamics calculations. They believe that this simulation approach may also be applicable to understanding bending behavior of other atomic monolayers. The article can be found on the Physical Review B website (I. Nikiforov, E. Dontsova, R.D. James, T. Dumitrica. Tight-binding theory of graphene bending. 2014. Physical Review B. 89, 155437).
Earlier research relating to this work appeared in a 2011 paper in Physical Review Letters (D.-B. Zhang, E. Akatyeva, T. Dumitrica. Bending ultrathin graphene at the margins of continuum mechanics. 2011. Physical Review Letters. 106, 255503). An article also appeared in MSI’s Research Bulletin (Understanding and Predicting Properties of Nanostructures: Insights From Atomic-level Simulations. Supercomputing Institute Research Bulletin, Spring 2010).
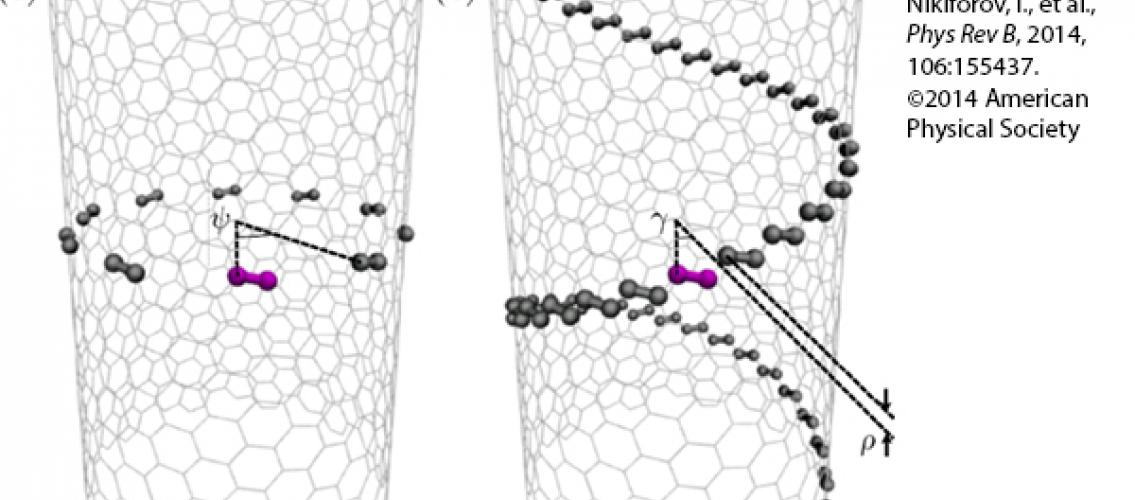
Image description: Schematic of the symmetries used in objective molecular dynamics simulations. The model simulated carbon nanotubes (CNTs) of varying diameter; these simulated ideal graphene sheets rolled into constant-curvature cylinders. (a) Pure rotation around the CNT axis of angle ψ. (b) Rotation around the CNT axis of angle γ combined with translation ρ along the CNT axis. Image and description Nikiforov, I., et al., Phys Rev B, 2014, 106:155437. ©2014 American Physical Society
posted on August 20, 2014.